The determinant of a 2-bridge knot

Given the continued fraction [a0,a1,...,an] = pn/qn    (with pn >= 0)  we have the recurrence relation [see eg Fowler's  book ]
pn  =  anpn-1  +  pn-2  (and similarly  qn  =  anqn-1  +  qn-2 - different initail conditions). This can be used to show
 det N(an,an-1,...,a0) = pn and here is the proof. The proof is easily modified to give the computation of the associated involutive quandle. The result is stated in the theorem below. Below we write xy or x^y instead of x*y. Involutive means (x*y)*y = x for any x and y in the quandle. Oriented links have associated quandles and unoriented links have associated involutive quandles.
 

2-generator involutive quandles

Given generators a and b it is easy to see that only 3 types of relations are possible a^(ba)m = b, a^(ba)m = a, and b^(ab)m = b.
No relations gives a quandle isomorphic to the infinite dihedral quandle Core(Z). This is Z with operation   rs  = 2s-r. It is also isomorphic to the conjugates of a and b in the free group on {a,b}:
  If we put in some relations we get a quotient quandle.

Theorem.  The first relation gives the dihedral quandle Core(Z2m+1)  - isomorphic to Z2m+1 generated by 0 and 1 with operation   rs  = 2s-r.   This is the involutive quandle of a 2-bridge knot with determinant 2m+1.

If we take the other two relations together we get the involutive quandle of a 2-bridge link with determinant 2m -  the dihedral quandle Core( Z2m).

The relation   a^(ba)m = a   alone gives a 3m element quandle  J3m  which contains  Zm  and  Z2m
and as a set is the disjoint union of these two quandles. In particular this is the quandle of an arc in B3
with a circle simply linked around it - 2m crossings.

Proof  We use the integer model of the free two generator involutive quandle.

We have for all k in Z,    1.  k0  = -k,    2.  k1  =  2-k,    3.  k01 =  k+2,    4.    k10  =  k-2.
Consider the (primary) relation   0 = n    By using 3 and 4 repeatedly we get 2k = n + 2k for all k.

Case i) n odd
From 1 we have 0 = -n    then   (n+2k+1) = -n + (n+2k+1)  ie 2k+1 = n + 2k+1 and so  k = n + k for all k. The result is then
Zn .

Case ii) n even
We now have to take into account the (secondary) relation k0  =  kn  for all k. So  k = 2n + k for all k. Add this to the relation derived above and we get. J3m   =  {r | r is even taken mod n or r is odd taken mod 2n} where n = 2m.

Notice that in case i) the secondary relation adds nothing as 2n+k = n+(n+k) = n+k = k already.

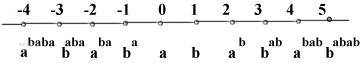
Example    J6    =  {0,1,2,3,5,7}
 


Question  Consider the functor from racks to  involutive quandles. What does the number of generators tell us? eg n-bridge links or n-braids have =< n generators.

Question  What about 3 generator involutive quandles? Can they be classified in some nice way? For example 0,1, i in the complex plane generate an involutive quandle. It is not free. What are the relations? An isomorphic quandle comes from generators (1,0,0), (0,1,0), (0,0,1) in 3-space.


Back to lecture 23    lecture 24     MA3F2 page     previous page     next page